publications

Books

2021

1. SpringerBriefs

   

   Non-Euclidean Laguerre Geometry and Incircular Nets

   Alexander I. Bobenko, Carl O. R. Lutz, Helmut Pottmann, and Jan Techter

   Springer , Cham, 2021

   TLDR Abs Bib arXiv HTML

   This textbook is a comprehensive and yet accessible introduction to non-Euclidean Laguerre geometry, for which there exists no previous systematic presentation in the literature. Moreover, we present new results by demonstrating all essential features of Laguerre geometry on the example of checkerboard incircular nets. Classical (Euclidean) Laguerre geometry studies oriented hyperplanes, oriented hyperspheres, and their oriented contact in Euclidean space. We describe how this can be generalized to arbitrary Cayley-Klein spaces, in particular hyperbolic and elliptic space, and study the corresponding groups of Laguerre transformations. We give an introduction to Lie geometry and describe how these Laguerre geometries can be obtained as subgeometries. As an application of two-dimensional Lie and Laguerre geometry we study the properties of checkerboard incircular nets.

   A comprehensive yet accessible introduction to non-Euclidean Laguerre geometry, complemented by a practical demonstration of all the essential features on the example of checkerboard circular nets.

   @book{BLP+2021,
     title = {Non-Euclidean Laguerre Geometry and Incircular Nets},
     author = {Bobenko, Alexander I. and Lutz, Carl O. R. and Pottmann, Helmut and Techter, Jan},
     year = {2021},
     series = {{{SpringerBriefs}} in {{Mathematics}}},
     publisher = {Springer},
     location = {Cham},
     doi = {10.1007/978-3-030-81847-0},
     isbn = {978-3-030-81846-3, 978-3-030-81847-0},
   }

Journal Articles

2024

1. IMRN

   

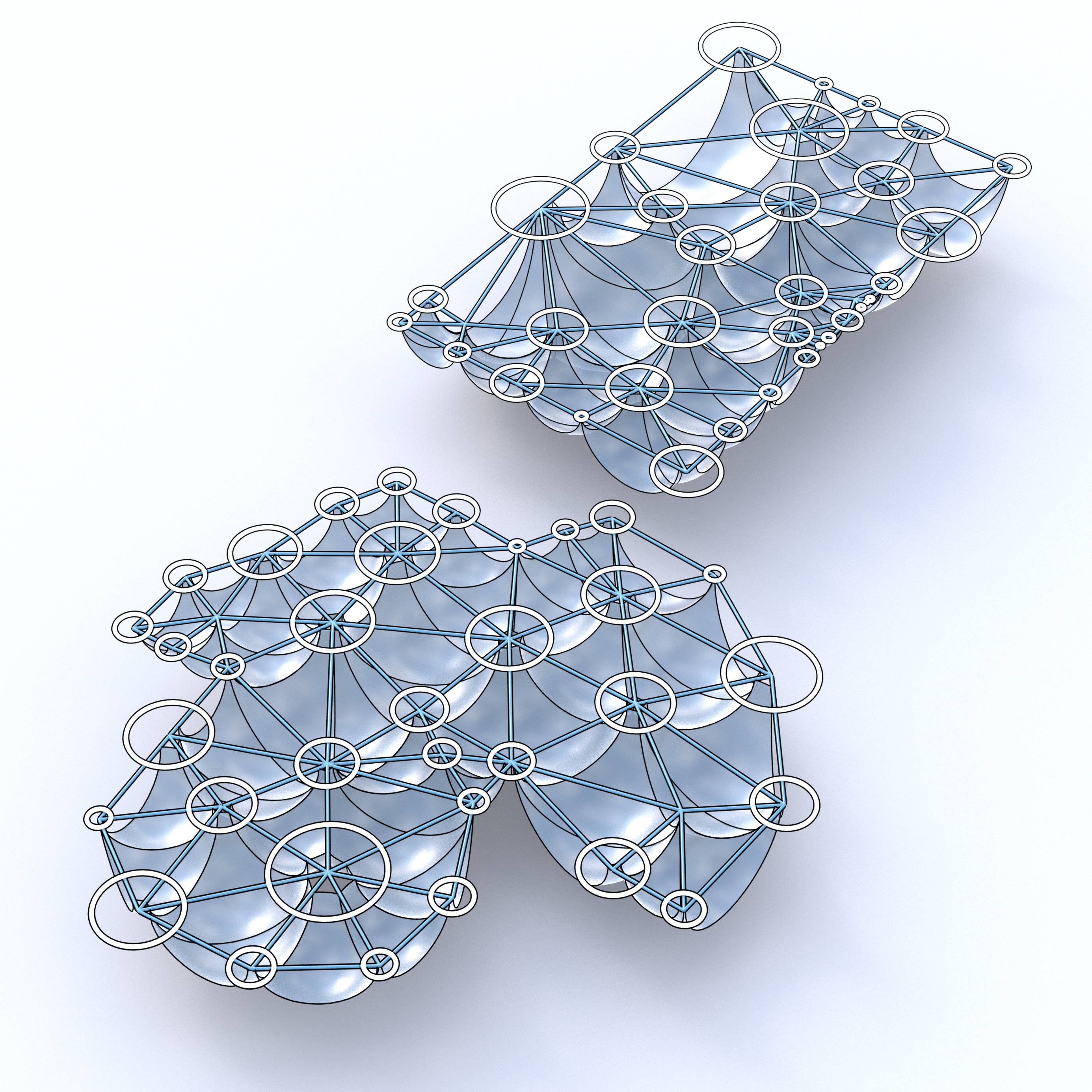   Decorated Discrete Conformal Maps and Convex Polyhedral Cusps

   Alexander I. Bobenko, and Carl O. R. Lutz

   International Mathematics Research Notices, Jun 2024

   TLDR Abs Bib arXiv HTML

   We discuss a notion of discrete conformal equivalence for decorated piecewise Euclidean surfaces (PE-surface), that is, PE-surfaces with a choice of circle about each vertex. It is closely related to inversive distance and hyperideal circle patterns. Under the assumption that the circles are non-intersecting, we prove the corresponding discrete uniformization theorem. The uniformization theorem for discrete conformal maps corresponds to the special case that all circles degenerate to points. Our proof relies on an intimate relationship between decorated PE-surfaces, canonical tessellations of hyperbolic surfaces and convex hyperbolic polyhedra. It is based on a concave variational principle, which also provides a method for the computation of decorated discrete conformal maps.

   We prove the discrete uniformization theorems for decorated PE surfaces and explore their relationship to hyperbolic polyhedra.

   @article{BL2024,
     title = {Decorated Discrete Conformal Maps and Convex Polyhedral Cusps},
     author = {Bobenko, Alexander I. and Lutz, Carl O. R.},
     year = {2024},
     month = jun,
     journal = {International Mathematics Research Notices},
     volume = {2024},
     issue = {12},
     pages = {9505-9534},
     doi = {10.1093/imrn/rnae016},
   }

2023

1. Geom Dedicata

   

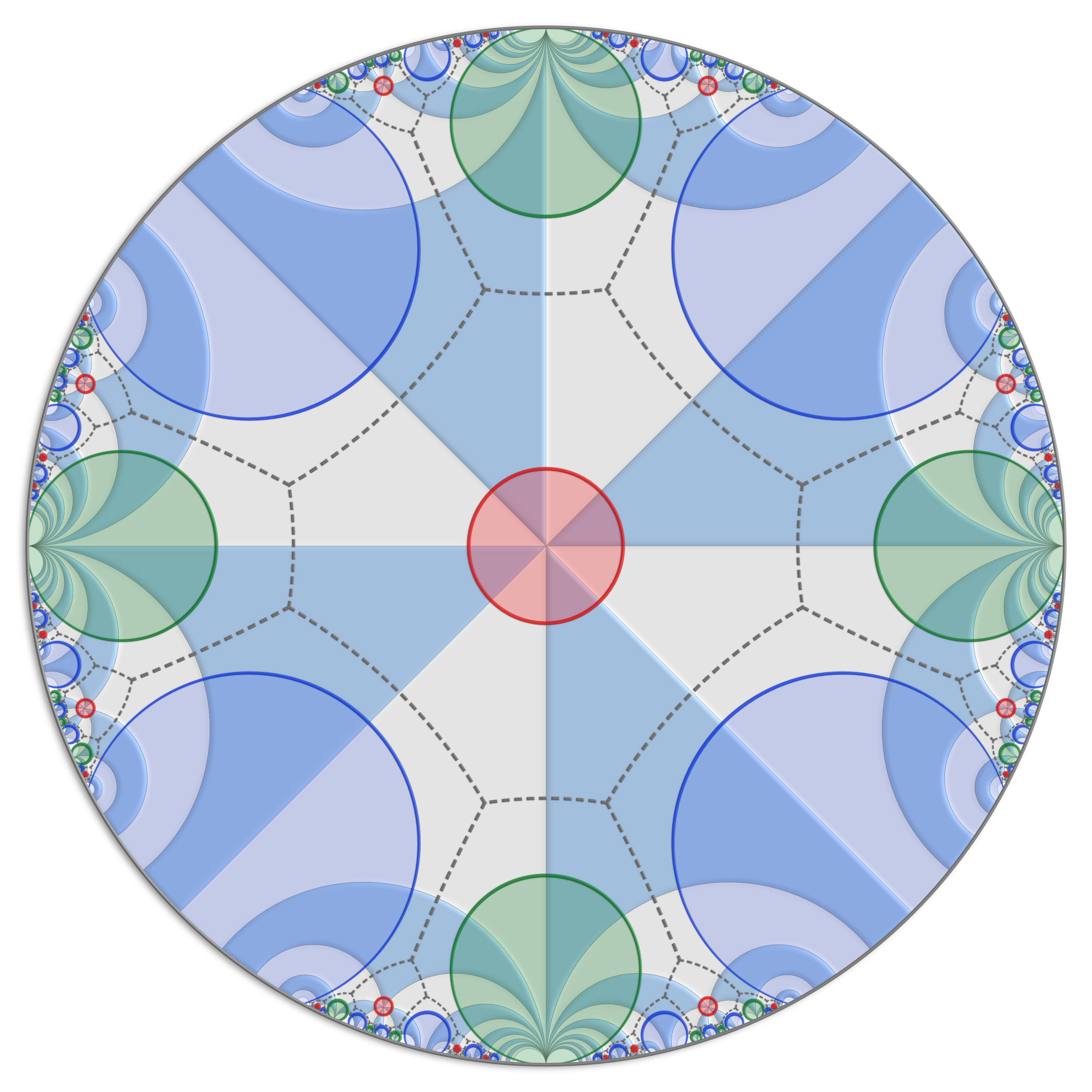   Canonical Tessellations of Decorated Hyperbolic Surfaces

   Carl O. R. Lutz

   Geometria Dedicata, Apr 2023

   TLDR Abs Bib arXiv HTML

   A decoration of a hyperbolic surface of finite type is a choice of circle, horocycle or hypercycle about each cone-point, cusp or flare of the surface, respectively. In this article we show that a decoration induces a unique canonical tessellation and dual decomposition of the underlying surface. They are analogues of the weighted Delaunay tessellation and Voronoi decomposition in the Euclidean plane. We develop a characterisation in terms of the hyperbolic geometric equivalents of DELAUNAY’s empty-discs and LAGUERRE’s tangent-distance, also known as power-distance. Furthermore, the relation between the tessellations and convex hulls in Minkowski space is presented, generalising the Epstein-Penner convex hull construction. This relation allows us to extend WEEKS’ flip algorithm to the case of decorated finite type hyperbolic surfaces. Finally, we give a simple description of the configuration space of decorations and show that any fixed hyperbolic surface only admits a finite number of combinatorially different canonical tessellations.

   We develop several characterizations of canonical tessellations of decorated hyperbolic surfaces and their configuration spaces.

   @article{Lutz2023,
     title = {Canonical Tessellations of Decorated Hyperbolic Surfaces},
     author = {Lutz, Carl O. R.},
     journal = {Geometria Dedicata},
     volume = {217},
     issue = {14},
     pages = {1-37},
     year = {2023},
     month = apr,
     publisher = {Springer},
     doi = {10.1007/s10711-022-00746-y},
   }

Theses

2024

1. Dr rer nat

   

   Decorated Discrete Conformal Equivalence, Canonical Tessellations, and Polyhedral Realization

   Carl O. R. Lutz

   TU Berlin , Berlin, Apr 2024

   TLDR Abs Bib HTML

   This cumulative dissertation introduces and develops the new theory of decorated discrete conformal equivalence. A decoration of a piecewise hyperbolic, Euclidean, or spherical surface is a choice of circle about each of its conical singularities. Our decorated surfaces are intimately related to inversive distance circle packings, canonical tessellations of hyperbolic surfaces, and convex hyperbolic polyhedra. We prove the discrete uniformization theorems for decorated surfaces.
   In the special case that all vertex circles degenerate to points, this recovers the known uniformization theorems for discrete conformal maps. Furthermore, we show that one can deform continuously between decorated piecewise hyperbolic, Euclidean, and spherical surfaces sharing the same fundamental discrete conformal invariant. Therefore, there is but one master theory of discrete conformal equivalence in different background geometries.
   Our approach is based on concave variational principles, which provide a way to compute the discrete uniformization and geometric transitions. They are given by the Legendre transformations of the volumes of convex hyperbolic polyhedra. Therefore, our discussion also provides an explicit variational method to compute the realization of hyperbolic surfaces with ends as the boundary of convex parabolic or Fuchsian polyhedra.
   A key aspect of the theory is to understand the combinatorial change that can accrue while continuously deforming a decorated discrete metric. We show that the combinatorics of decorated piecewise hyperbolic, Euclidean, and spherical surfaces correspond to the canonical tessellations of decorated hyperbolic surfaces with ends. Their decorated Teichmüller spaces are the configuration spaces of our variational principles. We analyze the decompositions of these spaces, which are induced by canonical tessellations. In particular, we generalize the Epstein-Penner convex hull construction, Weeks’ flip algorithm, and Akiyoshi’s compactification theorem to the case of hyperbolic surfaces with ends of infinite area.

   This cumulative dissertation introduces and develops the new theory of decorated discrete conformal equivalence. In particular, we prove the discrete uniformization theorems for decorated surfaces

   @thesis{Lutz2024,
     type = {phdthesis},
     title = {Decorated Discrete Conformal Equivalence, Canonical Tessellations, and Polyhedral Realization},
     author = {Lutz, Carl O. R.},
     year = {2024},
     school = {TU Berlin},
     location = {Berlin},
     pagetotal = {139},
     doi = {10.14279/depositonce-20357},
   }

preprint

2023

1. 

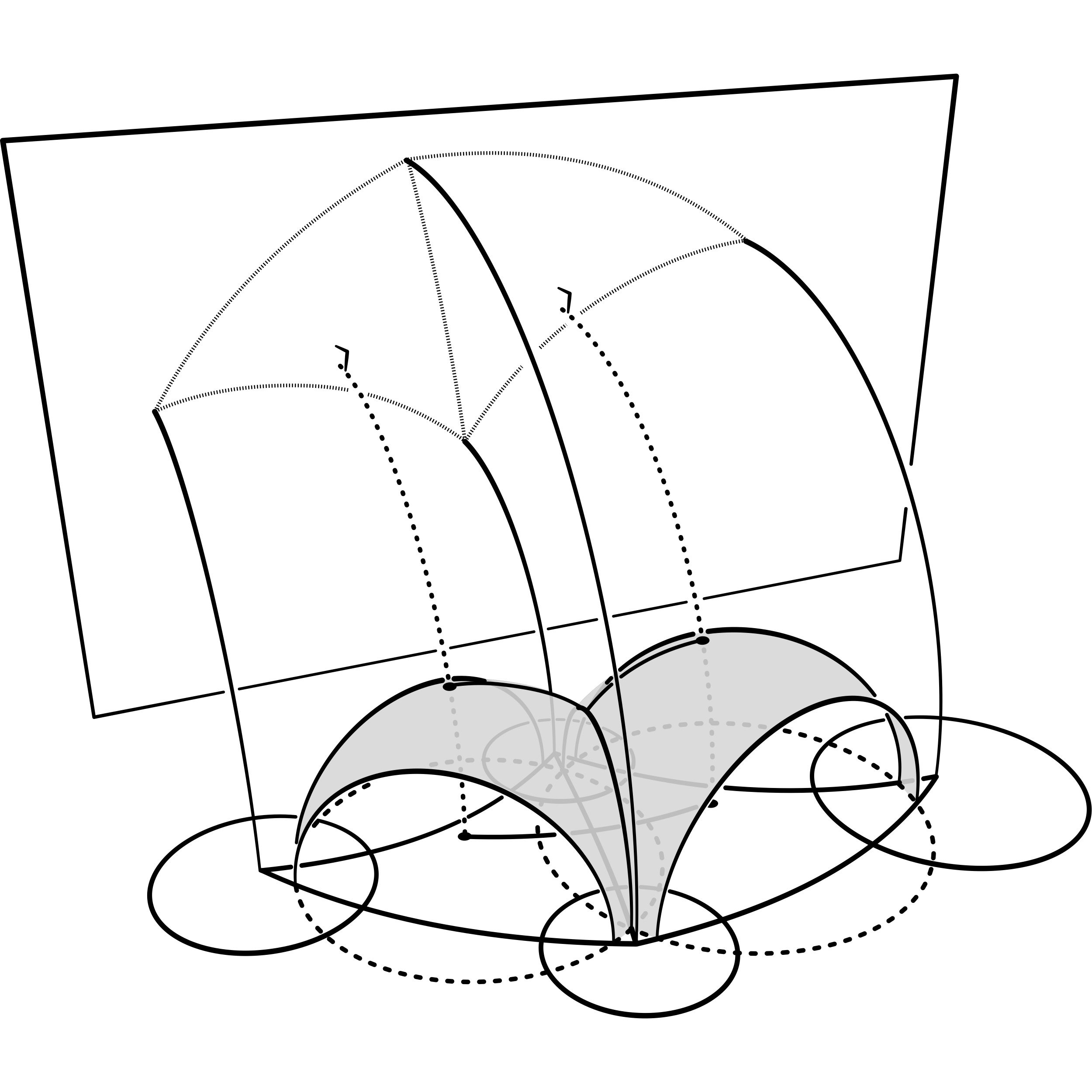   Decorated Discrete Conformal Equivalence in Non-Euclidean Geometries

   Alexander I. Bobenko, and Carl O. R. Lutz

   Oct 2023

   TLDR Abs Bib arXiv

   We introduce decorated piecewise hyperbolic and spherical surfaces and discuss their discrete conformal equivalence. A decoration is a choice of circle about each vertex of the surface. Our decorated surfaces are closely related to inversive distance circle packings, canonical tessellations of hyperbolic surfaces, and hyperbolic polyhedra.
   We prove the corresponding uniformization theorem. Furthermore, we show that on can deform continuously between decorated piecewise hyperbolic, Euclidean, and spherical surfaces sharing the same fundamental discrete conformal invariant. Therefore, there is one master theory of discrete conformal equivalence in different background geometries. Our approach is based on a variational principle, which also provides a way to compute the discrete uniformization and geometric transitions.

   We prove the discrete uniformization theorems for decorated piecewise hyperbolic and spherical surfaces and show that there is only one master theory of discrete conformal equivalence.

   @preprint{BL2023,
     title = {Decorated Discrete Conformal Equivalence in Non-Euclidean Geometries},
     author = {Bobenko, Alexander I. and Lutz, Carl O. R.},
     year = {2023},
     month = oct,
     eprint = {2310.17529},
     eprinttype = {arxiv},
     eprintclass = {math.GT},
   }